Author:
(1) CALLA TSCHANZ.
Table of Links
- Abstract and Intro
- Background on tropical perspective
- The expanded construction
- GIT stability
- Stack perspective
- The canonical moduli stack
- References
6. The canonical moduli stack
6.1 Properness and Deligne-Mumford property
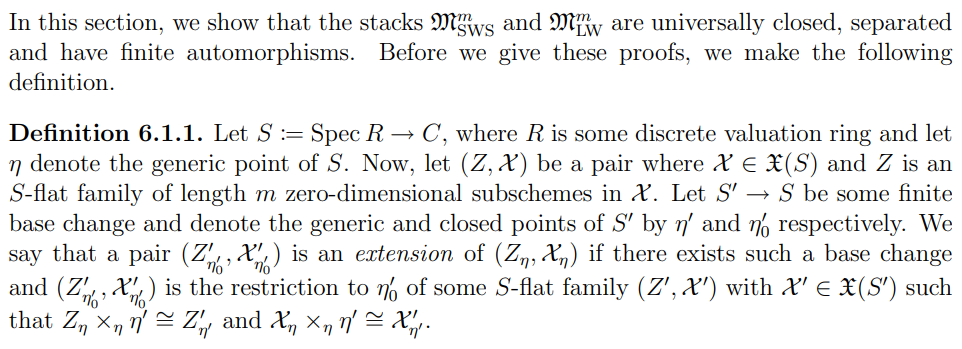
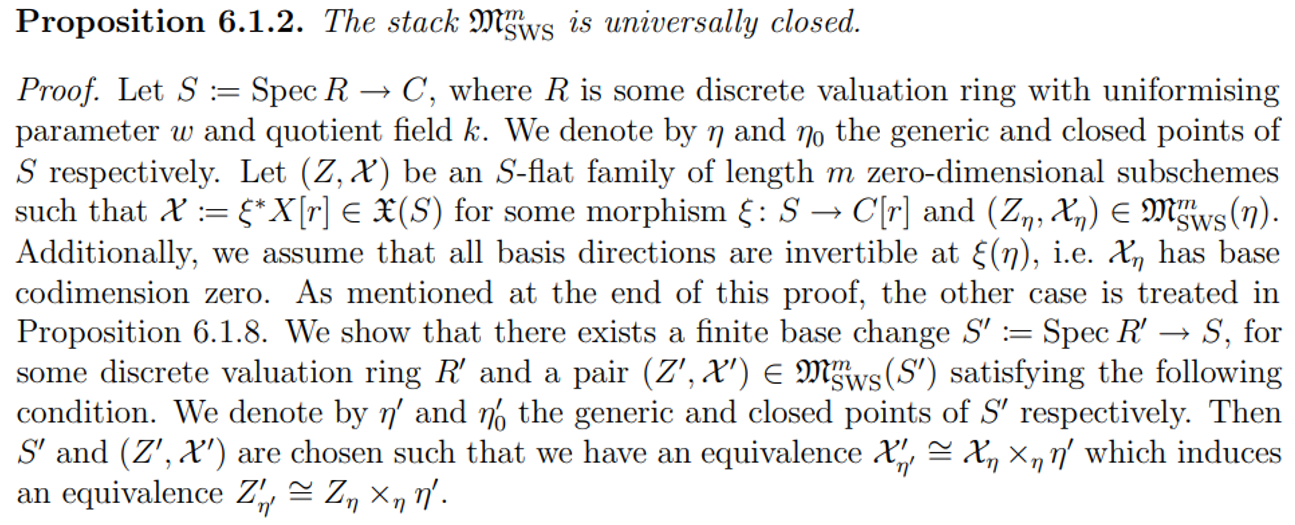

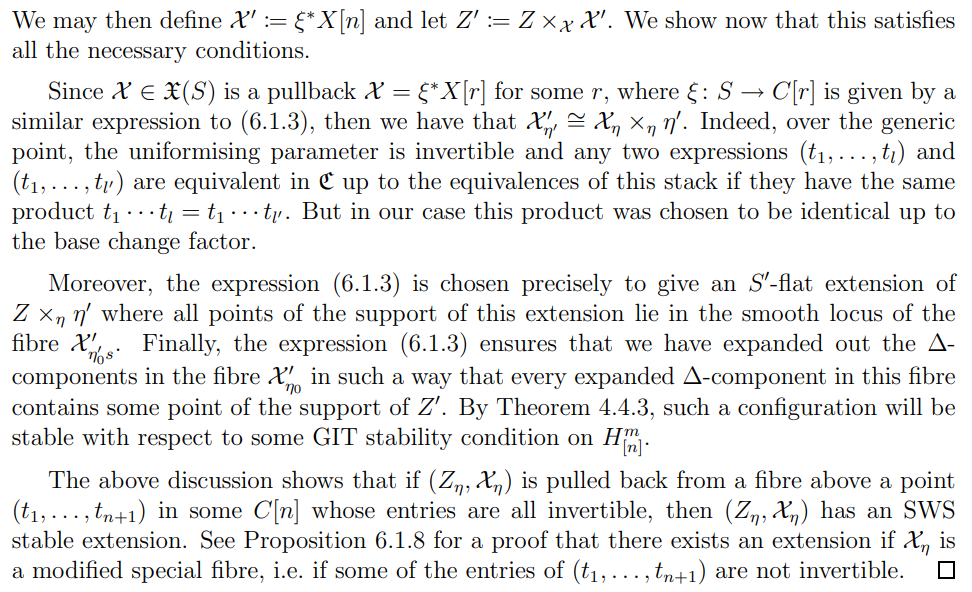
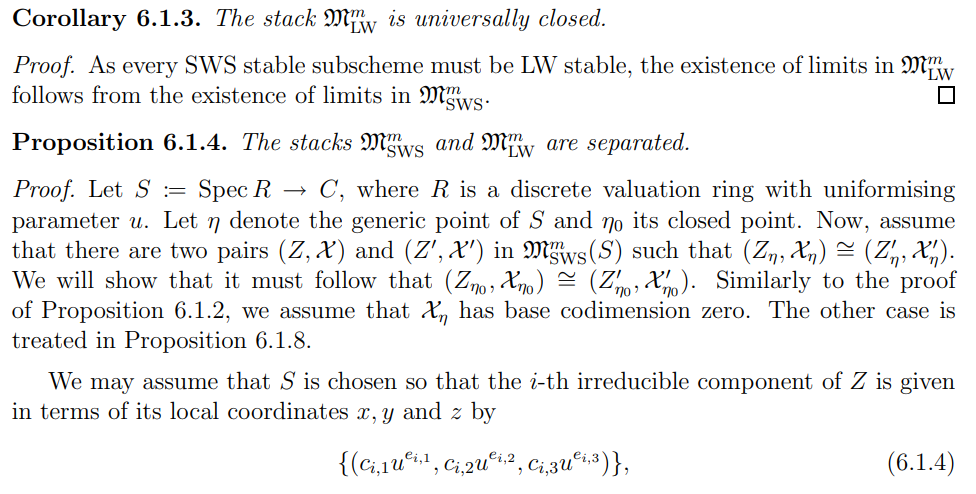
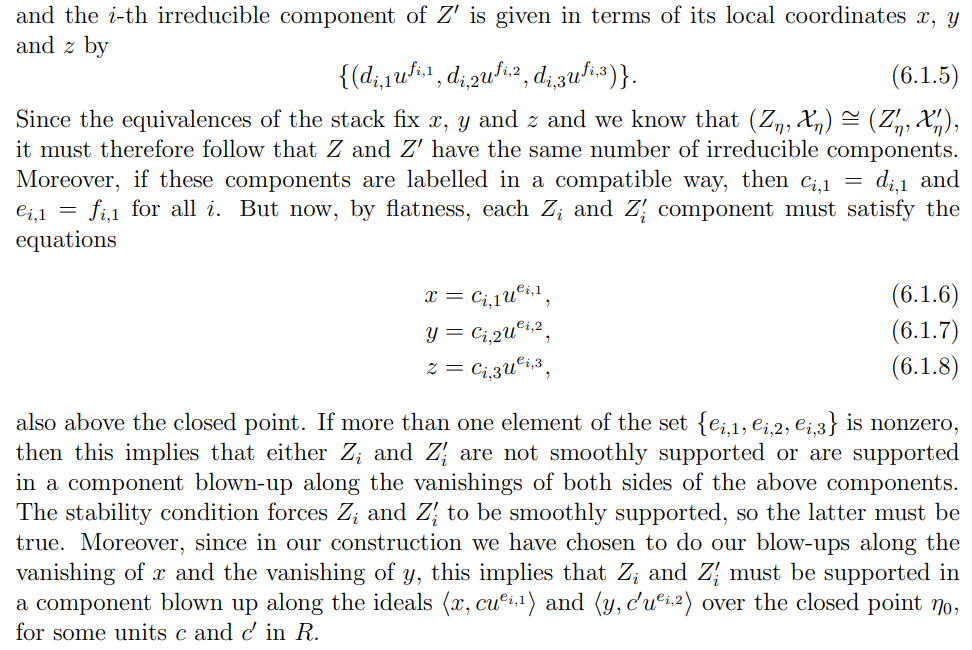
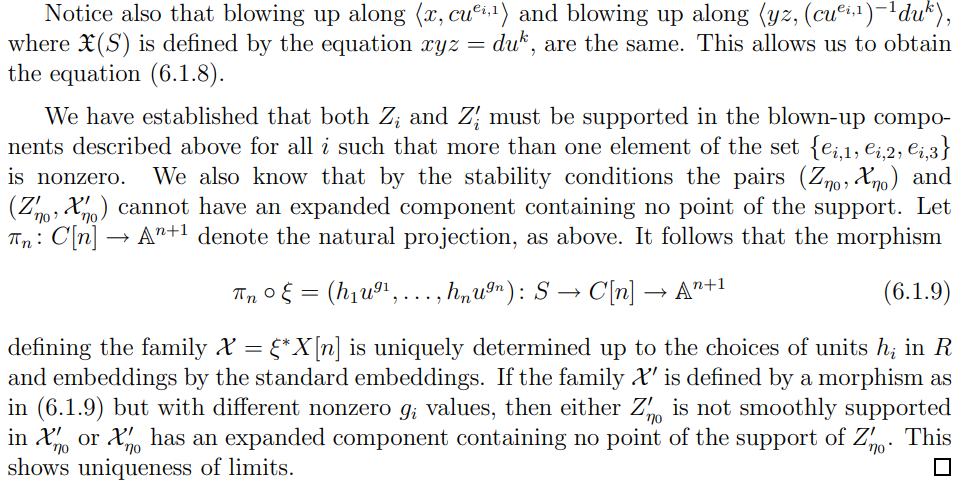
Existence and uniqueness of limits for special objects. We need to establish some definitions before we prove the following auxiliary result on existence and uniqueness of limits for special elements, i.e. when the fibre Xη over the generic point of S is a modified special fibre itself.
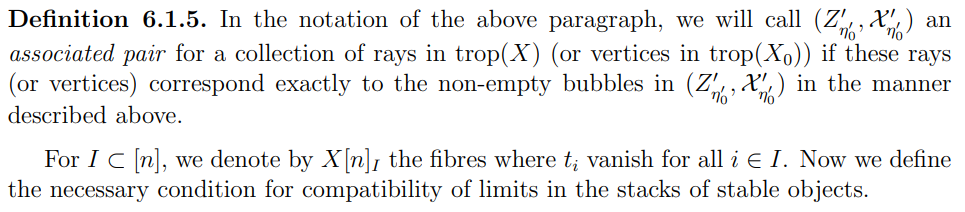

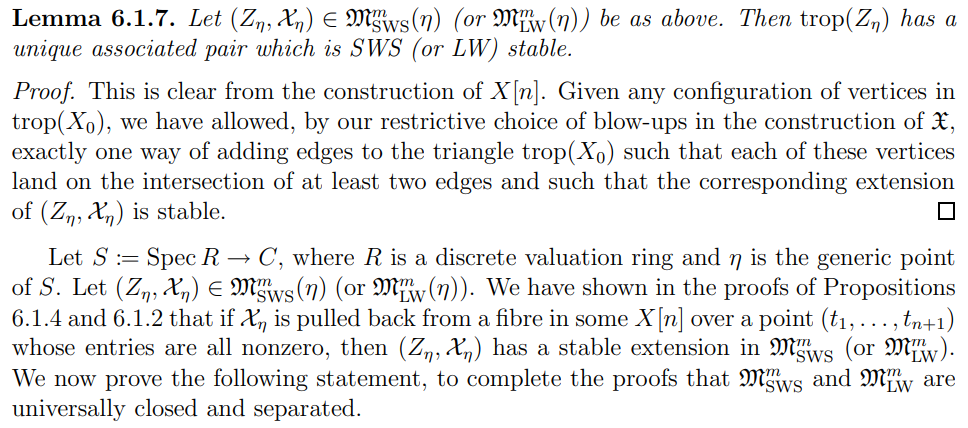
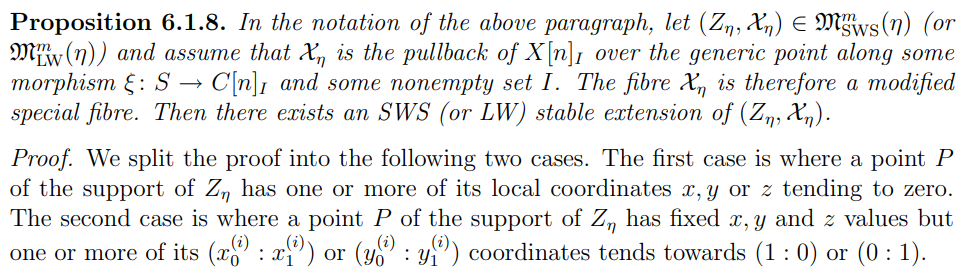
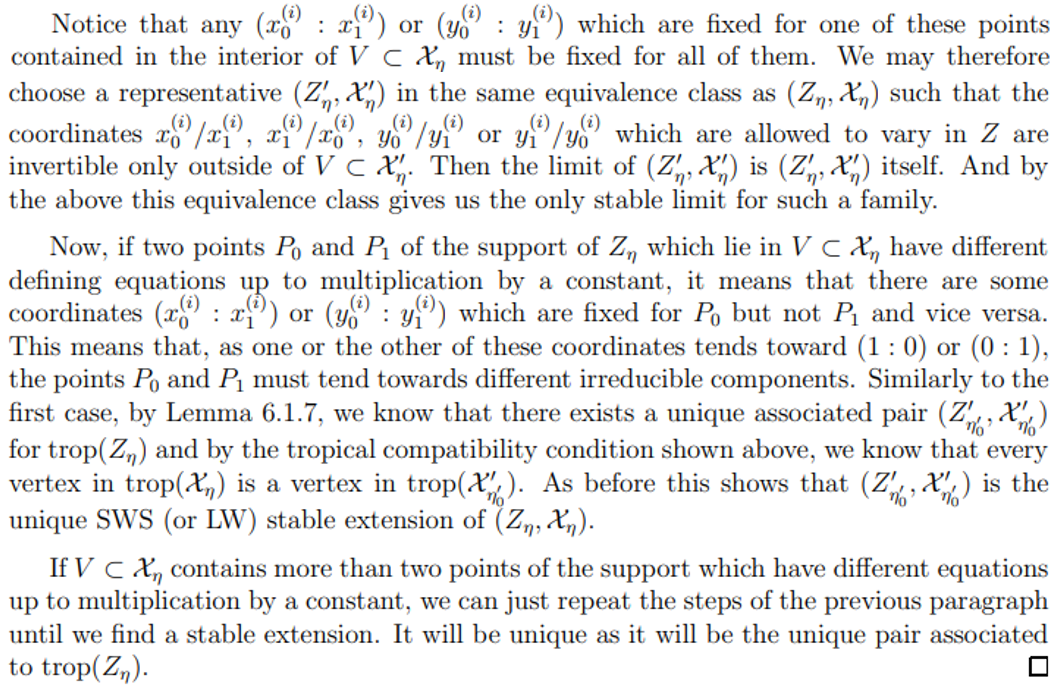
We start by proving existence and uniqueness of limits in the first case using the valuative criterion. Let V denote the irreducible component of Xη in the interior of which P lies. Notice that since P tends towards a codimension greater or equal to one stratum of X, then in order for its limit to be smoothly supported in an extension of (Zη, Xη), it will be necessary to expand out at least one ∆-component in this extension. There exists a smoothing from the interior of V in the fibre over the generic point to the interior of this expanded ∆-component in such an extension of (Zη, Xη) if and only if this ∆-component is equal to V in the fibre over the generic point. Moreover, if there is no such ∆-component equal to V, then none of the x, y or z coordinates can tend towards zero (because both sides of the defining equations must tend towards zero).

Deligne-Mumford property. Finally we show that both stacks of stable objects constructed have finite automorphisms.
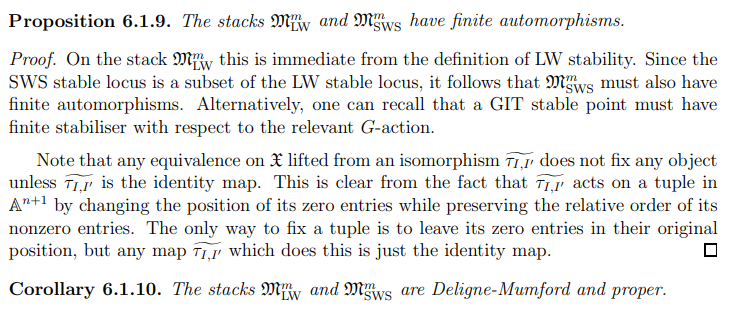
Proof. This follows directly from the results of this section.
6.2 An isomorphism of stacks
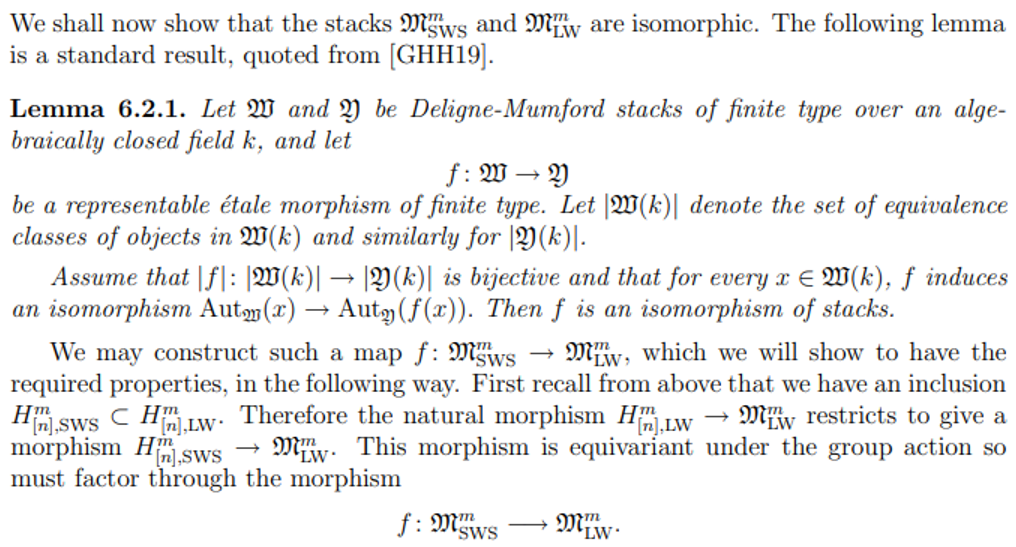
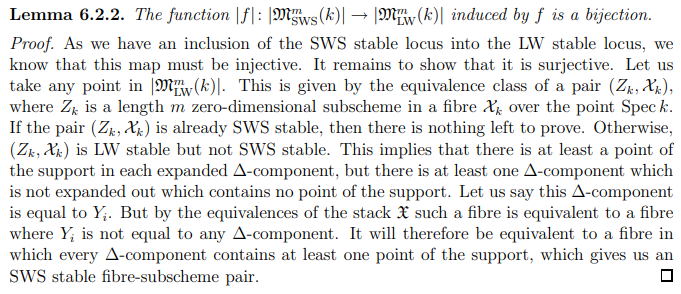
We will need also the following result from Alper and Kresch [AK16].

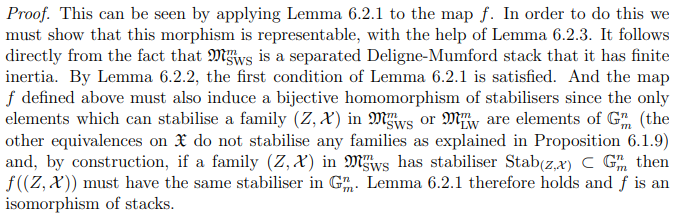
Now we are in a position to prove the following theorem:
This paper is available on arxiv under CC 4.0 license.

